Recently I saw some memes about the peculiar “sum”

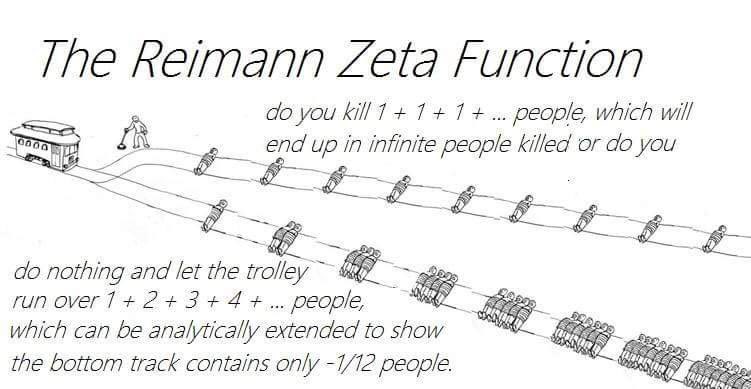
These memes are inspired by the Riemann zeta function
in which directly substituting $s=-1$ gives the $-\tfrac{1}{12}$ “sum” above.
Recently I saw some memes about the peculiar “sum”


These memes are inspired by the Riemann zeta function
in which directly substituting $s=-1$ gives the $-\tfrac{1}{12}$ “sum” above.
As a “365-day challenge,” I have been doing exercise every day for at least 15 minutes a day since last December, and will continue to do so throughout this year. I have to admit that this feels almost too easy.1 This is why I am in fact doing another challenge at the same time: to publish at least 40 blog posts this year.
Of course, I won’t publish anything of bad quality by my standard. So this challenge will definitely stretch me to learn and think more regularly, so that I can create something I am happy with every week or so.
On the other hand, publishing 40 articles doesn’t feel overwhelming, which leaves me enough room to work on research projects. A few years ago, I tried blogging on WeChat by setting too ambitious goals. I eventually stopped posting completely after a little more than 10 months; nevertheless, it was a rewarding experience.
With that, I am writing again this year. So far I have been enjoying it and have learned many new things already. Hopefully I won’t blow up my creative engine this time.
Lately, I have been listening to this podcast series that has completely changed how I see the history of science.
In the series, Viktor Blåsjö, a professor and an emerging historian of mathematics, told a mind-blowing story about Galileo which is nothing like what we are usually told about. We have been told by the teachers, by the media, and by the mainstream science philosophers, that Galileo was the “father of modern science.” But to the contrary, Galileo was not only poor in mathematics, but also a dilettante physicist. Let me name a few things from the podcast series and you will know how interesting it is:
When we first learned ordinary differential equations (ODEs) in class, we were told that the solution of
is $f(x)=Ce^x$ for an arbitrary constant $C$. We were told that this solution can be obtained either by observation and guessing (then it’s easy to verify solution), or by a separation of variable (e.g. integrate $dy/y=dx$). But these approaches don’t tell us what to do for higher-order equations, such as $f’'-3f’+2f=0$.
The operational calculus provides a convenient way to algebraically derive the solution to these linear ODEs. The derivation goes as follows.
We naturally avoid making mistakes. If you were a caveman who survives by hunting, you’d try not to make mistakes that could get you killed by animals.
But in the modern days, we no longer worry about ferocious animals. We now have much safer spaces that are tolerant of our mistakes. So we can afford to make more mistakes. In fact, making mistakes is how we learn.
Yet, people avoid mistakes because it makes you “look bad.” But there is no learning without making mistakes. So how can we gain the courage to learn without worrying about the mistakes we would make?
I think math has the answer.
When you begin to research a field of study, you often get overwhelmed by the amount of existing knowledge you have to learn before you could go further. One useful way to bootstrap yourself is to only learn a minimal amount of basic ideas that are enough for you to “survive” in the field. Such basic ideas are the Minimal Actionable Knowledge & Experience (MAKE) of the field. Here, I will try to present the “MAKE” of the field of fast matrix computations.
In the previous post I talked about anxiety caused by things that you actually have control over. This time I’d like to also touch on situations that you can’t really change, such as a pandemic.
A pandemic makes people anxious because it freezes life. A lot of activities have to be suspended. You can’t do what you would normally do. You don’t know how long the pandemic would last — probably a few months, probably a few years. All you can do is wait, indefinitely.
Some groups of people seem to be doing quite well in a lockdown situation. Mathematicians happen to be one such group.
Anxiety comes from desire. You are anxious when you desire something but not sure if you can get it.
I’d like to see different desires as being linearly independent.
Linearity. When you have many desires, they “superpose” to increase your level of anxiety. For example, if someone wants to be rich, be famous, to have a superb partner, and to be playing all day and not have to work … all at the same time, then this must be the most anxious person in the world.
Independence. Whether or not one desire is satisfied doesn’t affect your ability to satisfy another desire. For example, you don’t have to be rich to start doing things you like, you don’t have to be gorgeous to start building a good relationship, you don’t have to be the most intelligent person to be confident.
As a corollary, if you just give up most of your desires, you will stop being overly anxious and have much more energy to persue your most important desire.
80+ hours. I can’t remember the last time I have been without power at subzero temperature for this long.
Austin (and Texas) just went through a disaster. A winter storm devoured the city, turning it into a frozen world for a week. On Monday, January 15, 2021, temperature dropped below -10°C, coldest over the past century. Snow was thick enough to sink your entire boots in. While such cold weather is nothing special in northern states like Michigan, it is nothing the Texans have ever seen. The electricity infrastructures in Texas were absolutely unprepared for such extreme condition, leaving millions of people out of power throughout the week.
Unfortunately, I am one of the witnesses and sufferers in this historic event. To make things worse, both of the kitchen stove and hot water in my apartment rely on electricity, so we are out of every possible source of energy.
Long story short, we survived. And here is how.
“Being ignorant is bad.” So would most people think. I try hard to be more knowledgeable and I am still ashamed of my own ignorance oftentimes. But I am also learning more and more about the positive power of ignorance — a power that has been underestimated.